
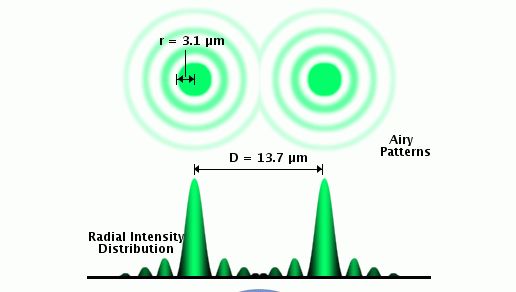
the star is then seen (in favourable circumstances of tranquil atmosphere, uniform temperature, etc.) as a perfectly round, well-defined planetary disc, surrounded by two, three, or more alternately dark and bright rings, which, if examined attentively, are seen to be slightly coloured at their borders. The disk and rings phenomenon had been known prior to Airy John Herschel described the appearance of a bright star seen through a telescope under high magnification for an 1828 article on light for the Encyclopedia Metropolitana: Both are named after George Biddell Airy.
#Diffraction limited telescope series
The diffraction pattern resulting from a uniformly illuminated, circular aperture has a bright central region, known as the Airy disk, which together with the series of concentric rings around is called the Airy pattern. The Airy disk is of importance in physics, optics, and astronomy. In optics, the Airy disk (or Airy disc) and Airy pattern are descriptions of the best- focused spot of light that a perfect lens with a circular aperture can make, limited by the diffraction of light. A real Airy disk created by passing a red laser beam through a 90- micrometre pinhole aperture with 27 orders of diffraction Airy disk captured by 2000 mm camera lens at f/25 aperture. Note that the red component is diffracted more than the blue, so that the center appears slightly bluish. A computer-generated Airy disk from diffracted white light ( D65 spectrum).

The grayscale intensities have been adjusted to enhance the brightness of the outer rings of the Airy pattern. A diffraction-limited laser beam, passed through diffraction-limited optics, will remain diffraction-limited, and will have a spatial or angular extent essentially equal to the resolution of the optics at the wavelength of the laser.Diffraction pattern in optics A computer-generated image of an Airy disk. The beam from a laser with near-ideal beam propagation properties may be described as being diffraction-limited.
#Diffraction limited telescope free
Space-based telescopes (such as Hubble, or a number of non-optical telescopes) always work at their diffraction limit, if their design is free of optical aberration. Radio telescopes are frequently diffraction-limited, because the wavelengths they use (from millimeters to meters) are so long that the atmospheric distortion is negligible.
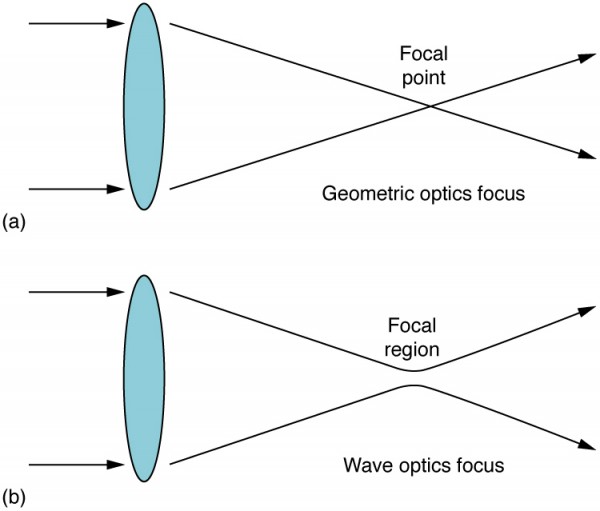
Advanced observatories have started using adaptive optics technology, resulting in greater image resolution for faint targets, but it is still difficult to reach the diffraction limit using adaptive optics. Optical telescopes on the Earth work at a much lower resolution than the diffraction limit because of the distortion introduced by the passage of light through several kilometres of turbulent atmosphere. However, most observations from Earth are seeing-limited due to atmospheric effects. In astronomy, a diffraction-limited observation is one that achieves the resolution of a theoretically ideal objective in the size of instrument used. At small apertures, such as f/22, most modern lenses are limited only by diffraction and not by aberrations or other imperfections in the construction.įor microscopic instruments, the diffraction-limited spatial resolution is proportional to the light wavelength, and to the numerical aperture of either the objective or the object illumination source, whichever is smaller. As one decreases the size of the aperture of a telescopic lens, diffraction proportionately increases. For telescopes with circular apertures, the size of the smallest feature in an image that is diffraction limited is the size of the Airy disk. The diffraction-limited angular resolution, in radians, of an instrument is proportional to the wavelength of the light being observed, and inversely proportional to the diameter of its objective's entrance aperture. For example, the blue star shows that the Hubble Space Telescope is almost diffraction-limited in the visible spectrum at 0.1 arcsecs, whereas the red circle shows that the human eye should have a resolving power of 20 arcsecs in theory, though normally only 60 arcsecs. Log-log plot of aperture diameter vs angular resolution at the diffraction limit for various light wavelengths compared with various astronomical instruments. Optical system with resolution performance at the instrument's theoretical limit Memorial to Ernst Karl Abbe, who approximated the diffraction limit of a microscope as d = λ 2 n sin θ, where d is the resolvable feature size, λ is the wavelength of light, n is the index of refraction of the medium being imaged in, and θ (depicted as α in the inscription) is the half-angle subtended by the optical objective lens (representing the numerical aperture).


 0 kommentar(er)
0 kommentar(er)
